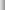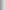In this paper we prove among others that, if A and B are positive definite matrices, then [the following inequality holds]:
0& \leq \int_{0}^{1}\left[ \det \left( \left( 1-t\right) A+tB\right) \right]
^{-1}dt-\left[ \det \left( \frac{A+B}{2}\right) \right] ^{-1} \\
& \leq \frac{1}{3}\left[ \frac{1}{2}\left( \left[ \det \left( A\right) %
\right] ^{-1}+\left[ \det \left( B\right) \right] ^{-1}\right) -\left[ \det
\left( \frac{A+B}{2}\right) \right] ^{-1}\right] \\
& \leq \frac{1}{2}\left( \left[ \det \left( A\right) \right] ^{-1}+\left[
\det \left( B\right) \right] ^{-1}\right) -\int_{0}^{1}\left[ \det \left(
\left( 1-t\right) A+tB\right) \right] ^{-1}dt \\
& \leq \frac{4}{3}\left[ \frac{1}{2}\left( \left[ \det \left( A\right) %
\right] ^{-1}+\left[ \det \left( B\right) \right] ^{-1}\right) -\left[ \det
\left( \frac{A+B}{2}\right) \right] ^{-1}\right] . |